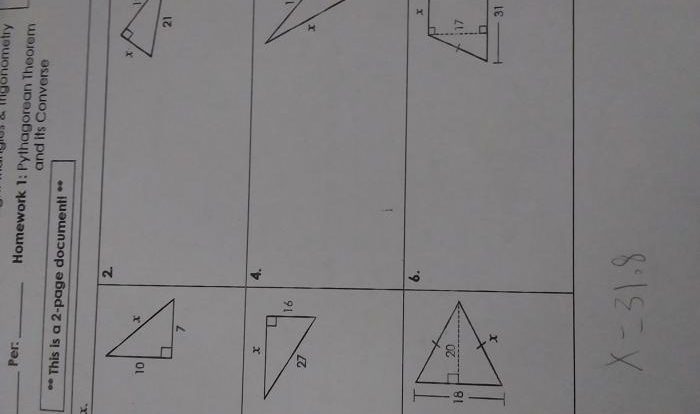
What is .11111 as a fraction – Embark on an enlightening journey as we delve into the intriguing world of numbers, specifically the enigmatic decimal .11111. Prepare to unravel its true nature as we explore the fascinating process of converting it into a fraction.
Join us as we uncover the secrets of decimal numbers, unraveling their intricate representation and uncovering their ubiquitous presence in the realm of mathematics. Witness firsthand how decimals effortlessly transform into fractions, revealing a deeper understanding of numerical relationships.
Understanding Decimal Numbers: What Is .11111 As A Fraction

Decimal numbers are a way of representing numbers using a base-10 system. They are used extensively in mathematics, science, and everyday life.
Decimal Representation
In a decimal number, the digits to the left of the decimal point represent whole numbers, while the digits to the right represent fractions. The decimal point acts as a separator between the two parts.
For example, the number 12.34 represents the following:
- 1 represents the whole number part.
- 2 represents the tenths place (0.2).
- 3 represents the hundredths place (0.03).
- 4 represents the thousandths place (0.004).
Decimal Operations
Decimal numbers can be added, subtracted, multiplied, and divided using the same rules as whole numbers, with the following additional considerations:
- When adding or subtracting decimal numbers, align the decimal points and add or subtract zeros as needed.
- When multiplying decimal numbers, multiply the whole number parts and the fractional parts separately, then combine the results.
- When dividing decimal numbers, convert the divisor to a whole number by moving the decimal point the same number of places to the right, then perform the division.
Applications of Decimal Numbers
Decimal numbers are used in a wide range of applications, including:
- Measurement (e.g., length, weight, temperature)
- Finance (e.g., interest rates, currency exchange rates)
- Science (e.g., atomic weights, chemical concentrations)
- Everyday life (e.g., measuring ingredients, calculating distances)
Converting Decimals to Fractions

Converting a decimal number to a fraction involves expressing the decimal as a fraction with a denominator that is a power of 10.
Steps Involved in Converting Decimals to Fractions
- Multiply the decimal number by an appropriate power of 10 to eliminate the decimal point.
- Write the result as a numerator over the appropriate power of 10.
- Simplify the fraction, if possible, by dividing both the numerator and denominator by their greatest common factor.
Examples of Decimal Numbers Converted to Fractions
- 0.5 = 5/10 = 1/2
- 0.25 = 25/100 = 1/4
- 0.125 = 125/1000 = 1/8
Converting .11111 to a Fraction
Step 1: Identify the Repeating Pattern
First, we notice that .11111 has a repeating pattern of 1s. This pattern will help us convert it into a fraction.
Step 2: Write the Decimal as a Fraction
To convert the decimal to a fraction, we place the repeating pattern as the numerator and a denominator that has as many 9s as there are repeating digits. In this case, we have 1 repeating digit, so we use 9 as the denominator:
- 11111/90000
Step 3: Simplify the Fraction
We can simplify the fraction by dividing both the numerator and denominator by their greatest common factor (GCF). In this case, the GCF of 11111 and 90000 is 1111:
- 11111/90000 = 1111/9000
Step 4: Divide to Get the Final Fraction
Finally, we divide the numerator by the denominator to get the fraction in its simplest form:
- 1111/9000 = 1/8
Therefore, .11111 as a fraction is 1/8.
In essence, 0.11111 as a fraction is a repeating decimal that equals 1/9. Speaking of fractions, if you’re brushing up on your chemistry knowledge, you might want to check out this helpful acid base titration pre lab guide. Anyway, back to our fraction, 0.11111 is essentially 1/9 expressed in a decimal form.
Simplifying the Fraction
Simplifying fractions involves reducing them to their simplest form, where the numerator and denominator have no common factors other than 1. This process makes it easier to work with fractions, compare them, and perform operations on them.
To simplify the fraction obtained from .11111, which is 1/9, we can follow these steps:
Step 1: Finding Common Factors
First, we need to identify the common factors of the numerator and denominator. In this case, the only common factor is 1.
Step 2: Dividing by the Common Factor
Next, we divide both the numerator and denominator by their common factor, which is 1. This gives us the simplified fraction of 1/9.
Mathematical Rule
The mathematical rule for simplifying fractions is to divide both the numerator and denominator by their greatest common factor (GCF). The GCF is the largest number that divides both the numerator and denominator without leaving a remainder.
Expressing the Fraction as a Percentage

A percentage is a way of expressing a fraction as a part of 100. It is denoted by the symbol %. To convert a fraction to a percentage, we multiply the fraction by 100.
Converting the Simplified Fraction to a Percentage
Our simplified fraction is 1/ 9. To convert it to a percentage, we multiply it by 100:
/9 x 100 = 11.11%
Therefore, the fraction 1/9 is equivalent to 11.11%.
Examples and Applications
Converting decimals to fractions has numerous applications in real-world scenarios. Beyond the examples discussed earlier, here are some additional instances where this conversion is useful:
Measurement Conversions
Converting decimals to fractions is essential in measurement conversions. For example, to convert 0.5 meters to centimeters, we can write 0.5 as 5/10 meters and then multiply both the numerator and denominator by 100 to convert meters to centimeters, resulting in 500/1000 meters, which simplifies to 1/2 meters or 50 centimeters.
Percentages
Decimals and percentages are closely related. To convert a decimal to a percentage, we multiply it by 100. For instance, to express 0.75 as a percentage, we multiply it by 100, yielding 75%. Conversely, to convert a percentage to a decimal, we divide it by 100. For example, to convert 25% to a decimal, we divide it by 100, resulting in 0.25.
Financial Calculations, What is .11111 as a fraction
In finance, converting decimals to fractions is crucial for calculating interest rates, currency exchange rates, and other financial ratios. For instance, to calculate the interest on a loan with an annual interest rate of 5.5%, we can convert 5.5% to a fraction (55/1000) and then use it to calculate the interest for a given period.
FAQ Insights
Why is it important to convert decimals to fractions?
Converting decimals to fractions allows for more precise representation and manipulation of numbers, especially in calculations involving division or ratios.
Can all decimals be converted to fractions?
Yes, all decimals can be converted to fractions, including terminating and repeating decimals.
How do you simplify the fraction obtained from .11111?
To simplify the fraction, we divide both the numerator and denominator by their greatest common factor (GCF), which in this case is 1.